by Mathew Barlow, UML Professor of Climate Science
1. Definitions
Vorticity is a measure of local rotation in the atmosphere, and potential vorticity is an extension of vorticity that further accounts for the distribution of mass relative to the rotation. Potential vorticity can be thought of as the fluid equivalent to angular momentum. It may sound somewhat technical and esoteric but has a straightforward physical interpretation, as we’ll see below, and directly relates to many interesting atmospheric phenomena. Potential vorticity is also a very useful quantity to consider for the ocean but here we will just focus on the atmosphere.
(For more introductory discussion of vorticity, including the role of Earth’s rotation, see: https://www.weather.gov/jetstream/vort_max .)
2. Physical Interpretation
To keep things relatively simple, let’s start with a standard example: a spinning ice skater.
https://www.youtube.com/watch?v=VmeM0BNnGR0
An ice skater can spin faster or slower by moving their arms in or out. Put another way, they can rotate faster or slower by moving more of their mass closer to or farther away from their axis of rotation. While their rotation is not constant depending on how their mass is distributed, there is a rotation-related quantity that does remain constant: their angular momentum, which accounts both for speed of rotation and distribution of mass.
Air acts in a similar way: a rotating column of air will rotate faster if it is stretched into a narrower column and rotate slower if it is squashed into a wider column.
Like the ice skater, the rotation of the column can increase or decrease depending on whether its mass is distributed closer or farther from the axis of rotation, but there is a quantity related to rotation that stays constant, accounting for both rotation and mass distribution. For the ice skater, the constant quantity is angular momentum; for the column of air, the constant quantity is potential vorticity.
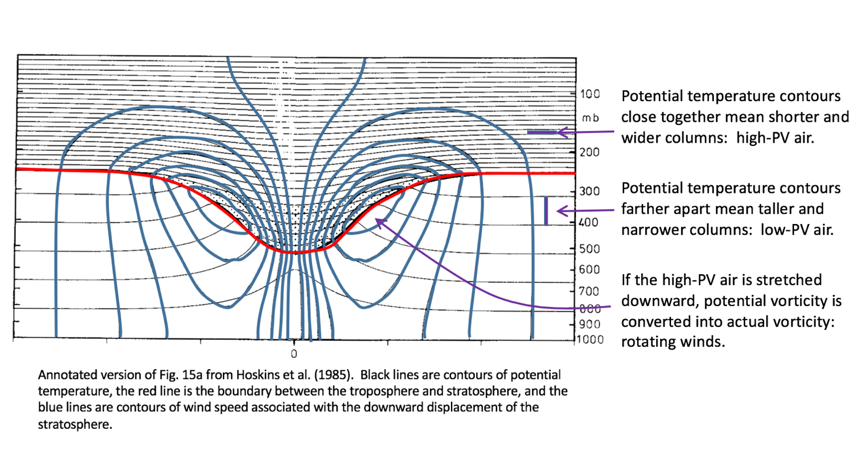
If we define the height of the column as the distance between between two values of potential temperature (θ), as in the figure above, the height of the column is directly related to the horizontal distribution of mass and the potential vorticity can be represented as the ratio of the vorticity to the height of the column. Since the potential vorticity stays constant, there is always a relationship between the vorticity and the height of the column to keep the ratio the same: increase the height of the column, which stretches it thin, and the rotation increases; decrease the height of the column, which squashes it wide, and the rotation decreases. Having a specific definition for potential vorticity allows us to quantify this association and understand the exact relationship between column height and rate of rotation.
This is the “potential” part of potential vorticity: the wider the column, the more potential to increase vorticity if the column is stretched. If you want to look directly at rotation, you look at vorticity; if you want to look at the potential for rotation, you look at potential vorticity. We consider why you might want to do that in section 4.
Technical Note 1: To fully consider the rotation of a column of air, you have to include the contribution from Earth’s rotation. The term we use for vorticity when including the effects of Earth’s rotation is “absolute vorticity,” otherwise we use “relative vorticity.” Potential vorticity is the absolute vorticity divided by the height of the column.
Technical Note 2: Potential vorticity is only constant in the absence of “diabatic heating.” Diabatic heating (and cooling) is caused by factors such as the condensation and evaporation of water, and radiative processes. The largest source of diabatic heating in the troposphere is the latent heat released during the condensation associated with heavy precipitation. Diabatic heating does not include the heating and cooling associated with expansion and compression of air as it rises and sinks; that is referred to as “adiabatic heating.”
3. Relationship to stability
The definition of column height in terms of potential temperature highlights the relationship between potential vorticity and static stability. Static stability is defined as the change in potential temperature with height: the faster the potential temperature increases with height, the more stable the atmosphere is. Where potential temperature is increasing rapidly with height, two given values of potential temperature (defining the height of a column) will be closer together than where potential temperature is increasing slowly. So column heights are shorter where stability is high and column heights are taller where stability is low: there is an inverse relationship between stability and column heights. Accordingly, we can think of potential vorticity as the ratio of vorticity to column height or, equivalently, as the product of vorticity and stability.
Areas of high stability, therefore, are areas with relatively short and wide columns of air that have high potential for creating vorticity, if the air columns can be stretched. Much of the rotating winds at mid and upper-levels in winter storms are produced by stretching very stable stratospheric air downward, as seen in the following figure:
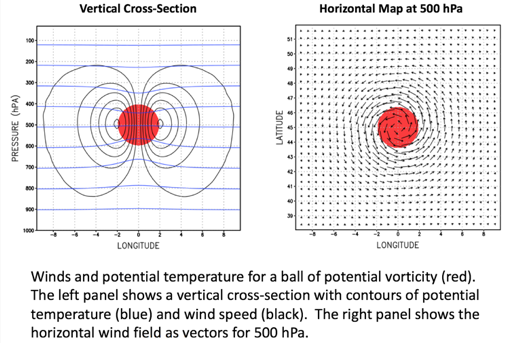
For a simpler perspective, we can also consider an isolated spherical ball of potential vorticity:
Note that the ball of potential vorticity is associated with both relative vorticity (rotation in the wind field) and with changes to static stability (potential temperature contours are closer together inside the ball and farther apart above and below it).
Technical note 3: To provide an easy-to-deal-with field, potential vorticity is usually defined in terms of a column of infinitesimal diameter and height, so that the column height (or stability) is written in terms of a differential of potential temperature.
4. Who cares?
Potential vorticity has a number of properties that make it useful to examine:
•In the absence of diabatic heating, it is a conserved quantity away from the surface. That is, if we follow a little piece of air around, its potential vorticity never changes if there is no diabatic heating. If large changes are observed, that signals significant diabatic activity.
•“One variable to rule them all.” Most other variables can be approximately calculated from potential vorticity (except for moisture and diabatically-forced flow). That is, if you know the three-dimensional distribution of potential vorticity, you can use it to calculate the large-scale structure of the three-dimensional wind field, temperature field, and pressure field. So potential vorticity is sort of a summary, one-stop-shopping variable.
•Conversion of potential vorticity to actual vorticity is an important part of many interesting meteorological situations, including winter storms.
•Areas where potential vorticity changes rapidly are important to the movement of large-scale atmospheric waves.
•It has its own theme song: https://www.youtube.com/watch?v=g2-RpvGGQU4 . Okay, maybe not useful, exactly, but fun in a geeky kind of way.
5. To read further
Potential vorticity is covered in all of the standard atmospheric dynamics textbooks. For most topics, I find it useful to read multiple authors – the differing perspectives give me more opportunities to sort things out.
Some textbooks that I have found helpful for understanding potential vorticity include the following:
Martin, J.E., 2013. Mid-latitude atmospheric dynamics: a first course. John Wiley & Sons.
Lackmann, G., 2011. Midlatitude synoptic meteorology: dynamics, analysis, and forecasting (p. 345). Boston: American Meteorological Society.
Holton, J.R. and Hakim, G.J., 2012. An introduction to dynamic meteorology (Vol. 88). Academic press.
Bluestein, H. B., 1993: Synoptic-dynamic meteorology in midlatitudes. Volume II: Observations and theory of weather systems. Oxford University Press.
Vallis, G.K., 2017. Atmospheric and oceanic fluid dynamics. 2nd Edition. Cambridge University Press.
There are also several papers that discuss potential vorticity from a general perspective, including:
Hoskins, B. J., M. McIntyre, and A. Robertson, 1985: On the use and significance of isentropic potential vorticity maps. Quart. J. Roy. Meteor. Soc., 111, 877-946.
Morgan, M. C., and J. W. Nielsen-Gammon, 1998: Using tropopause maps to diagnose midlatitude weather systems. Mon. Wea. Rev., 126, 2555-2579.
Brennan, M.J., Lackmann, G.M. and Mahoney, K.M., 2008. Potential vorticity (PV) thinking in operations: The utility of nonconservation. Weather and Forecasting, 23(1), pp.168-182.
Acknowledgements
This discussion is part of outreach efforts for NSF AGS-1657921.