by Mathew Barlow, UML Professor of Climate Science
This is a brief discussion of some of the ways different isolated areas of vorticity – vortices – can interact with each other and how those interactions are relevant to the atmosphere. (And ocean, but that’s for another time.)
Vorticity is a local measure of rotation: if you dropped a pinwheel or rotor into the flow at a point, whether or not the pinwheel or rotor turned would tell you whether there was any local rotation.
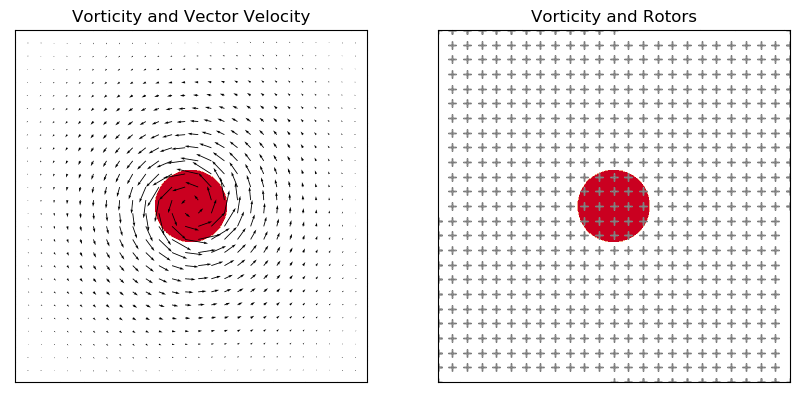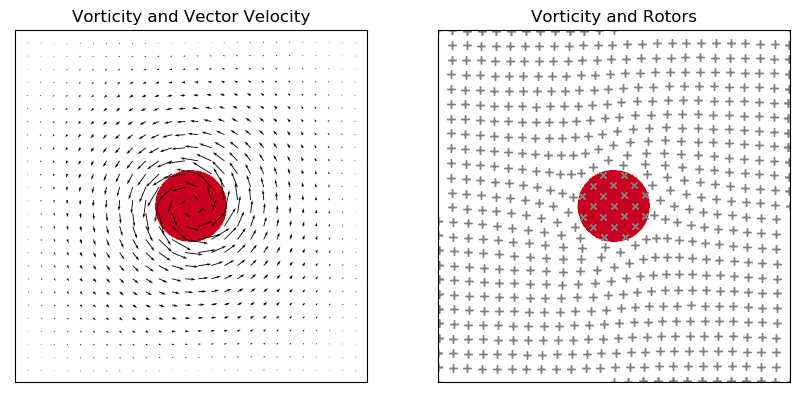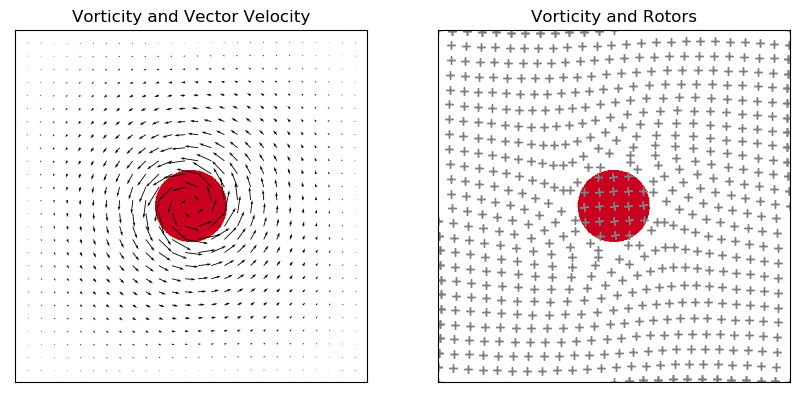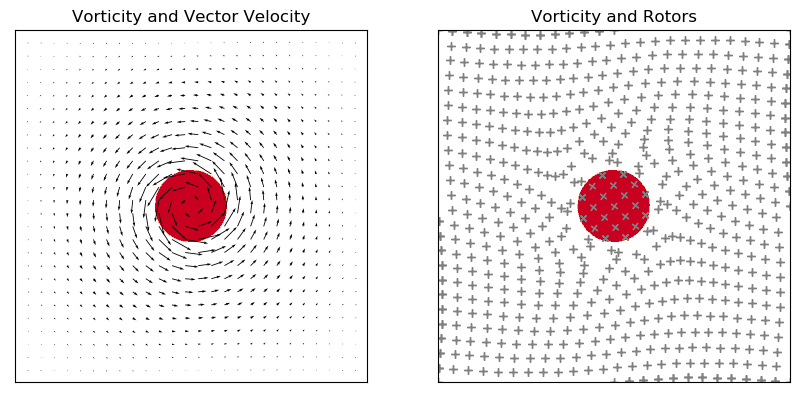
The wind field associated with an isolated area of vorticity extends out beyond the vorticity. Here’s an example for a disk of constant vorticity (a Rankine vortex). Red is constant positive vorticity, white is zero vorticity.
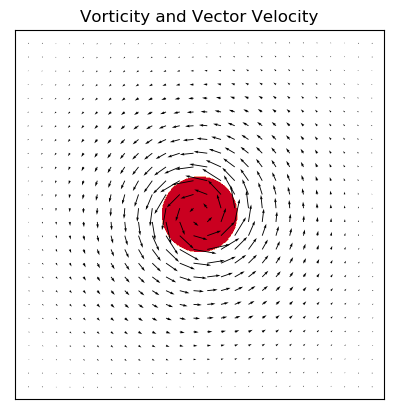
In the area of vorticity (red), the air is locally rotating; everywhere else the air is moving but not locally rotating. Here is a visualization that includes little rotors (‘+’ marks) to visualize the local rotation. Note that outside the disk of vorticity the rotors are moving but not turning whereas inside the disk of vorticity the rotors are moving and turning. Inside the vorticity area, the rotors are maintaining the same relationship to each other – this is called “solid body rotation” since that part of the fluid is moving like a solid.
So if isolated areas of vorticity (vortices) are close to each other, they can interact as the wind field of each moves (advects) the vorticity of the other. The interactions can take a variety of forms depending on relative strength, size, and motion of the two vortices.
For two vortices rotating in the same direction, the simplest case is the two orbiting each other. (This effect does not require the rotation of the Earth; this idealized case has f=0.)
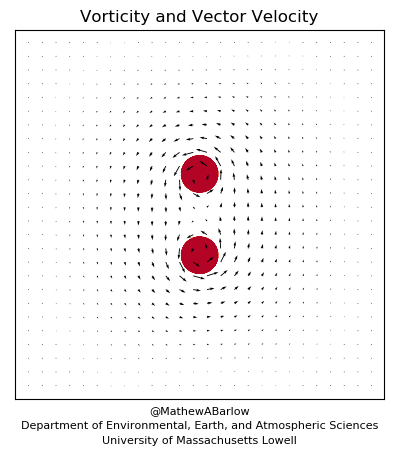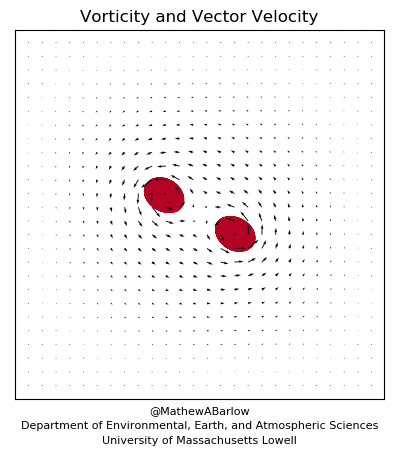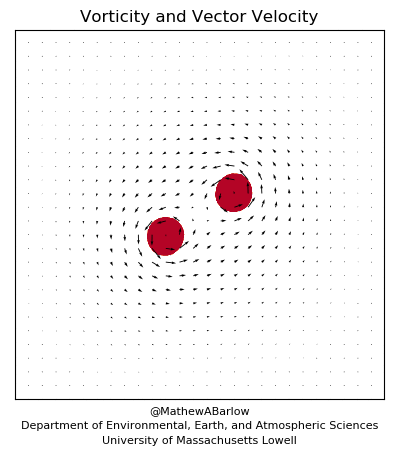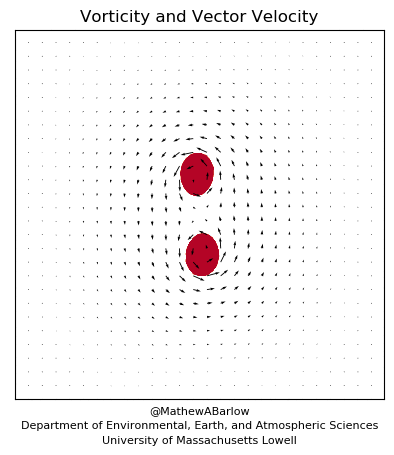
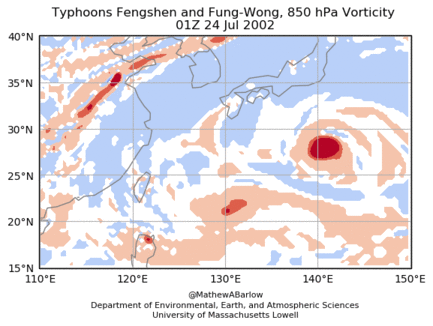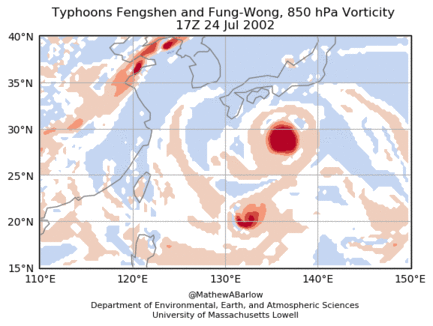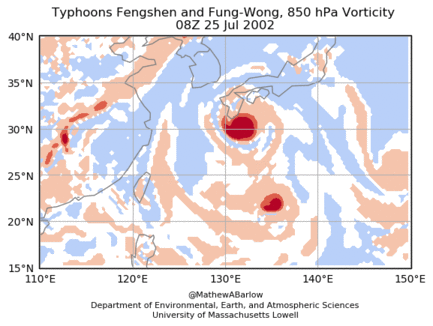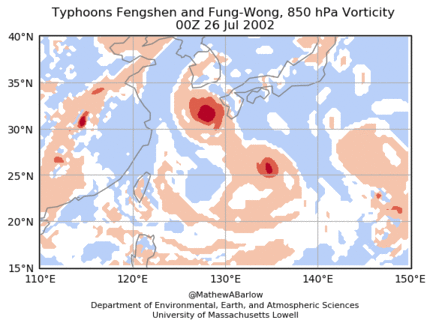
Does this happen in the atmosphere? Yes, most clearly when tropical cyclones come close to each other. This interaction is often called the “Fujiwhara effect.” Here’s an example with two different-sized typhoons.
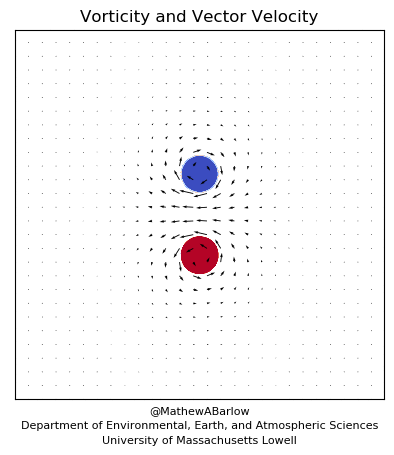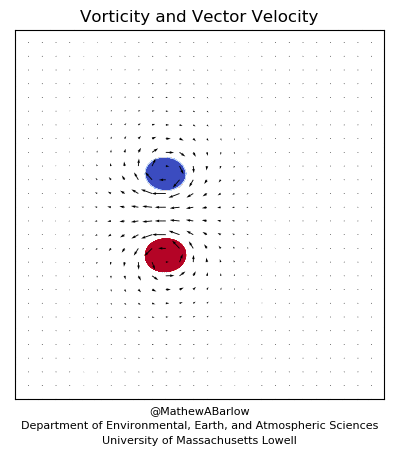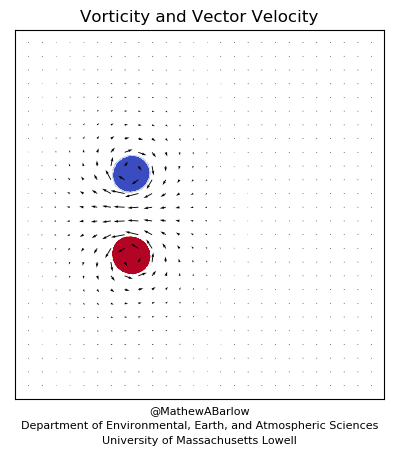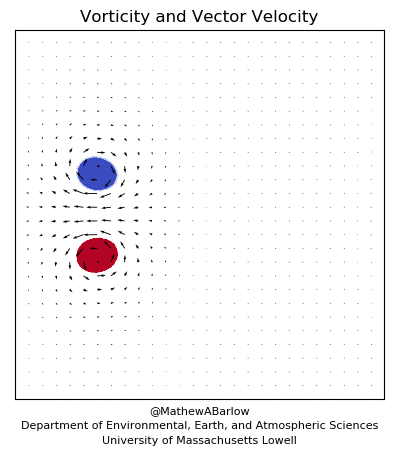
For two vortices rotating in opposite directions, the simplest interaction is for them to move in lockstep, with positive vorticity north of negative vorticity (+/-) moving eastward and negative vorticity north of positive vorticity (-/+) moving westward:
Isolated positive-negative pairs don’t tend to occur that often in large-scale atmospheric flow, but they can occur embedded within the westerlies. A negative-north / positive south pair in the westerlies can lead to a Rex block and a positive-north / negative-south pair can be realized as a jet streak. In a Rex block, the westward movement of the pair opposes the westerlies and can lead to a stationary blocking feature, whereas in a jet streak, the eastward movement of the pair outpaces the westerlies, leading to a feature that moves through the westerly flow.
In addition to these specific cases, this type of thinking can be applied quite generally, because any vorticity field, no matter how complicated, can be thought of as the sum of many individual vortices – in a similar way to how a digital image is comprised of individual pixels.
Moreover, all the cases here are for purely two-dimensional flow with no rotation of the Earth, for simplicity, but can be generalized to account for the rotation of the Earth by considering absolute vorticity instead of relative vorticity, and to account for 3D flow by considering potential vorticity.
For a brief introduction to potential vorticity, see: https://storm.uml.edu/~metweb/Blog/?p=330
Notes
Animations made using a modified version of Geoff Vallis’ barotropic vorticity program: http://empslocal.ex.ac.uk/people/staff/gv219/codes/index.html…
To keep things as simple as possible, f is set to zero in all cases.
Further Reading
Dritschel, D.G. and Waugh, D.W., 1992. Quantification of the inelastic interaction of unequal vortices in two‐dimensional vortex dynamics. Physics of Fluids A: Fluid Dynamics, 4(8), pp.1737-1744.
Fujiwhara, S, 1921: The natural tendency towards symmetry of motion and its application as a principle of motion. QJRMS, 47, 287-293.
Leweke, T., Le Dizes, S. and Williamson, C.H., 2016: Dynamics and instabilities of vortex pairs. Annual Review of Fluid Mechanics, 48, 507-541.
